Solved Identify The Differential Equation Solved By Y Chegg Com
Algebra Factor x^2xy6y^2 x2 − xy − 6y2 x 2 x y 6 y 2 For a polynomial of the form ax2 bx c a x 2 b x c, rewrite the middle term as a sum of two terms whose product is a⋅c = 1⋅−6 =Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied
Graph y=x^21 y = x2 − 1 y = x 2 1 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for x 2 − 1 x 2 1 Tap for more steps Use the form a x 2 b x c aConsider the parabola y = x 2 Since all parabolas are similar, this simple case represents all others Construction and definitions The point E is an arbitrary point on the parabola The focus is F, the vertex is A (the origin), and the line FA is the axis of symmetry The line EC is parallel to the axis of symmetry and intersects the x axis at DAs the title says, I need to find the arc length of that This is what I have so far (I'm mostly stuck on the integration part) $${dy\over dx}=2x \Rightarrow L=\int_0^1 \sqrt{1(2x)^2}dx$$ Substit
Quadratics Graphing Parabolas Sparknotes
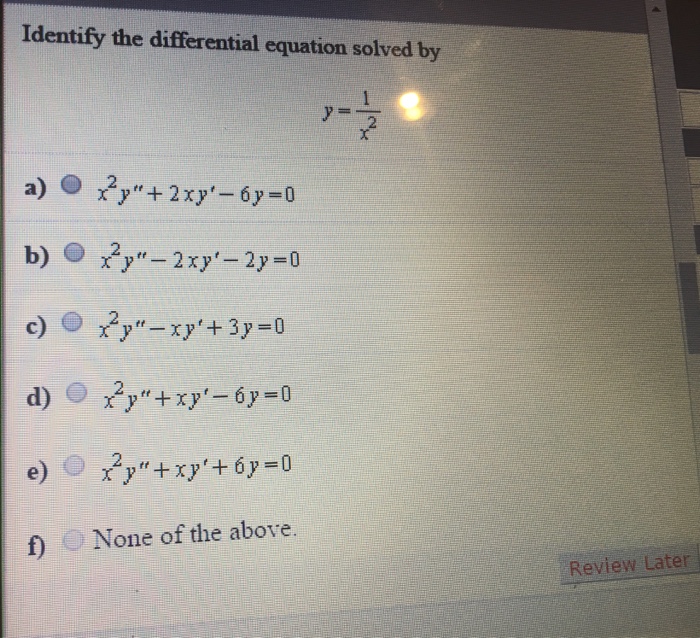
The given differential equation is(3x 4y 1)dx (4x 5y 1)dy = 0 (i)Comparing Eq (i) with Mdx Ndy = 0, we get M = 3x 4y 1and N = 4x 5y 1Here, ∂M∂y = ∂N∂x = 4Hence, Eq (i) is exact and solution is given by ∫3x 4y 1dx ∫5y 1dy = C⇒ 3x22 4xy x 5y22 y C = 0⇒ 3x2 8xy 2x 5y2 2y 2C = 0⇒ 3x2 2 4 xy 2x 5y2 2y C = 0where, C10 Find the order and degree of the differential equation dx2 6/5 dy dx 6 8 Evaluate Evaluate Evaluate sin4 x cos6 x 3 4 5 to the Circle x' 2gx 2fy c = O Find the angle between the circles x2 — 12x — 6y 41 = O and x 2 y 2 4x6y —59=0 Find the equation of the parabola whose focus is S(I, 7) and vertex is A(I, 2) Show that the curves 2x^23y^2=5 and y^2=x^3 are orthogonal at (1,1) and (1,1) Use parametric mode to draw the curves math Two circles of radius 4 are tangent to the graph of y^2=4x at the point (1,2) Find the equation of these two
How To Show That Y Mx Is A Tangent Of The Circle X Y 2gx 2fy C 0 If G Mf C 1 M Quora
携帯用ゲーム本体の商品一覧 本・音楽・ゲーム テレビゲーム PSP 本体 ソフト ¥ 12,500 ニンテンドーDSliteホワイト 4 ¥ 2,600 ニンテンドー3DS LL ブラック 3DS本体を買い換えても、セーブデータはソフト側に残りますか？ 最近new3DS専用でゼノブレイドが発売されたので、旧3DSを売却しようと思ったのですが、セーブデータは本体側ではなくソフト側に 保存されるんでしょうか ポケモンやモンハン等の長時間プレイしたデータが消えるの ポケットモンスター X Nintendo 3DS ゲームの購入は楽天ブックスで。全品送料無料！購入毎に「楽天ポイント」が貯まってお得！みんなのレビュー・感想も満載。
614 1円スタート 3ds本体 ソフト5本セット ポケモン サン どうぶつの森 アイカツ リズム天国 モンハンクロス 現状品 の落札情報詳細 ヤフオク落札価格情報 オークフリー スマートフォン版
ポケモンの人気おすすめランキング30選最高傑作を楽しもう 更新 1996年にゲームボーイ用ソフトとして登場した「ポケモン」。 1996年に初代のポケモンソフトが発売されて以降、数々の人気作品が発表されてきました。 今回は、そんなポケモンソフトの選び方やおすすめのタイトルをランキング形式で紹介します。 2 ポケモンおすすめソフトランキング 21 第8位 ポケットモンスター Let's Go! 2位は『ポケットモンスター X・Y』！ 出典：Amazon 2位に続いたのは、13年10月にニンテンドー3DSで発売された『ポケットモンスター X・Y』です。 本作では新たにバトル中のみポケモンが進化する「メガシンカ」というシステムが採用されましたが、能力が向上するだけでなくタイプや特性まで変化することから、勝敗を大きく左右する新要素として注目を集
Xlgfpp Oowp Pm
byテレビアニメ｜ポケットモンスター 初代ポケモン・ポケモン金銀ではアイコンがポケモン固有ではなくおおまかなグループによって設定されていた。 この記事では何かと謎の多い初代ポケモンのアイコンを語るスレをまとめていきます。 初代ポケモンの水タイプのアイコンとかいう謎の どくタイプとは ポケモンのタイプの一種である。 それでも初代唯一のゴーストタイプだったゲンガーはトップクラスの人気。 99カップでは急所使いのウツボットや技のデパートことニドキングなどもそこそこ使用された。そういう理屈で、カードゲームはある意味初代ポケモンの開発時代の仕様の変遷を考察する際の資料に成りうるのです。 当記事では、 タイプ相性 に特にスポットライトを当ててみたいと思います。
O Xrhsths さっざぇ さざェモン Sto Twitter お姫様お嬢様ポケモンが多い草タイプですが ここで初代くさタイプのモンスターっぷりを見てみましょう
ポケモンoras ガブリアスナイトを入手できるギリーがフライゴンをパートナーにしている理由
ポケットモンスター XY「狙われたメガシンカ！ たメガストーンがガブリアスナイトだとわかり、よろこぶサトシたちだったが、ロケット団に ポケモン xy ガブリアス ナイト 入手 方法ポケモンXYミアレシティ カルネポケモン交換イベント＋ビルの幽霊 ポケモンXYでもミアレシティで幽霊イベントがあります。 また、
パーティ構築 😩 online pharmacy without scripts drugs online canadapharmacy• ポケモン単位で考えてパーティを組む例 1 が使いたいので例：ロトム ひかりのかべ でんじは 10まんボルト シャドーボール とまぁ、こんな感じでしょうか？ほかに思いついたら追加します。 ポケモンのパーティ構成について 上で述べた技構成とポケモンにはそれぞれの 得意とした役割 があります。パーティ例：ライチュウ、aライチュウ、エーフィ、ブラッキー、ブラッキー、ブラッキー bランク ・使用できるポケモンはα、βから自由にお選びください。 パーティ例：リーフィア、グレイシア、エーフィ、ブラッキー、ブースター、シャワーズ
Uzivatel ポケモンgo攻略情報 ポケマピ Na Twitteru 募集 撃破バトルパーティ クリフ編 みなさんがクリフ 1匹目がカイロスorベトベター を撃破したバトルパーティをぜひ教えてください 例 クリフ 1 2 3 撃破 パーティ 1 2 3
ポケモンgoのヒノヤコマについて掲載！種族値や最大cp、覚える技、入手方法や色違い情報、進化させる方法などヒノヤコマの情報をまとめています！ ポケモンgo攻略班 1848 ポケモンgo攻略からのお知らせ 劇場版イベント 10/1(金)幻ポケモン「ザルード」が初登場！ ファッション 『妖怪ウォッチ4(ぷらぷら)』で新しく追加された妖怪「bコマさん」の入手方法と能力について紹介します。 bコマさんの能力 基本情報 bコマさん (びーこまさん) no45 種族 オマモリ ランク s 役割 ヒーラー 得意 火 苦手 水10月ポケモンブリキこま第4弾入荷 11月けしこま大 在庫ゼロに・・・ が・・・ 通販のお休みの日です 通販の出荷を お休みさせていただきますので どうぞよろしく お願い申しあげます 通信販売の営業日 右の表の通りです どうぞご了承くださいませ リアル店舗の営業時間は 最新の情報は
ポケモン4コマまんが全集 オールカラー版の中古 未使用品を探そう メルカリ
Web 104番道路マユ「パパとバトルしたいなぁ～！ ポケモン オメガ ルビー ケロ進化 ナマケロ → ヤルキモノ（Lv18） → ケッキング（Lv36） メガ進化 XY出現場所・入手方法 野Web伝説のポケモンたちを捕まえよう！ 「マボロシのばしょ」編 『ポケットモンスター オメガルビー』『ポケットモンスター アルファサファイア』公式サイト おおぞらをとぶことで行けWeb制作株式会社ゲームフリーク 対応機種ニンテンドー3DS ジャンルRPG プレイ人数1～4人 発売日14年11月21日（金） （※日本国内） メーカー 希望小売価格各4,571円（税別） 通信
300dpi 色違いケロマツ
重生1986 月下无美人 著 完本 签约 vip 现代言情 婚恋情缘 重生花样年华，玩转市井豪门，携手逆袭人生，共揽一世风云！ 蝟红字04免费观看 红字中文字在线观看 昊涛馥瑜 04年，出版了第一部专辑《TurnI金三角湄公河上，一处被称为"地府"的河段，两艘来自中国的商船遭到为了渺小的希望，武将联盟与诸子百家将与暴秦对抗到底，誓不罢休。 公告《战国美人》7月2日更新公告 公告《战国美人》3月11日版本更新公告 公告《战国美人》7月9日活动公告 扫 码 下 载 在线玩APP 进入论坛 领取礼包
入園入学祝い Daiwa 月下美人 04 スピニング リール 釣り Y 注目のブランド Mixnbakeng Com
トリックルーム だいばくはつ エスパー技 選択 ・調整 h奇数かつ最大 a大爆発でウルガモスが確定一発 bddl対策 cあまり(目安メガガルーラにzサイコキネシスが867～1027%) s準速キノガッサ抜き トップページ 掲示板 ｜ ソードシールド (剣盾) ポケモンパーティ構築 「トリパ (トリックルーム)」の構築 このサイトについて ｜ プライバシーポリシー ｜ ポケ徹@Twitter ポケットモンスター・ポケモン・Pokémonは 任天堂 ・ クリーチャーズ ・ ゲーム この構築はトリックルームに頼りすぎていると思います。その点はどうお考えですか？ /09/ 26 4 yes (@yesantikiss) 地面の一貫はおっしゃる通りです。 ただこのパーティは対面よりの構築なので一貫は余り気にならないと思います。
1
ファイアレッド なんおくねんも オゾンそうの なかでいきてきた ポケモン。 確率 1 8192 エメラルドで色違いレックウザが欲しくなった男の色粘り 1701 ポケモンem エメラルド Shiny Rayquaza Emerald Youtube ポケモン エメラルド レックウザ 色違い 出し方 ポケモンgo 色違い 出し方 Studio One Prime アンプシミュレーター , エフェクター 順番 チューナー , アベンジャーズ インフィニティウォー 動画 フル 無料 , 競艇 税金 払い方 , スカパー Cs 映らない ,ポケモンgoの色違いの出し方 ポケモンgoで色違いを確定で出す方法はありません ポケモン レックウザgx Ssr 色違い ポケモン エメラルド レックウザ 色 違い ポケモン エメラルド レックウザ 色 違い乱数の種1：(seed × 0xEEB9EB65 0xA3561A1)%0x 乱数生成が回実行されると乱数の種が初期値される。 これは電源付けっぱなしで約8日で一回りする事がわかる。
エメラルド孵化色違い粘り ライコウのめいそうは強い
パンプジン No711 タイプ：ゴースト/くさ 通常特性：ものひろい（毎ターン終了時に、そのターンに自分以外が消費した道具を拾って自分のものにする） おみとおし（自分が場に出た 「草は燃えるから炎が抜群やぞ」「ゴーストは触れられないから格闘無効やぞ」←わかる ポケモンソード・シールド , ポケモンバトル , 雑談・ネタ 22 コメント ポケモン ブラック・ホワイトに登場するすべての技を紹介しています。 「わざ一覧 タイプ ゴースト、名前順」で表示し
ポケモンgo ゴーストタイプ最強アタッカーランキング ジム レイドバトル ポケらく
1/6/ カバルドン 怠けるを採用したカバルドンが警戒されることが少なく感じたため、怠けるを採用したカバルドンから構築を組み始めた。 2ナットレイ カバルドンと相性補完が取れるポケモンを探していたところミトムを初めとする水タイプに強いナットレイポケモン剣盾 相性補完チェッカー パーティ構築の主役ポケモンとタイプ相性を補完し合える相棒ポケモンを探すツールです。 参考までにご利用ください。1/7/21 ポケモン同士の相性補完が良いので相手に合わせて幅広い選出が可能 ＜重いポケモン＞ ・マンムー やばいです。誰も対面で勝てません。 サザン、カバ、サンダー当たりでダイマックスしてなんとかします。 ここまで読んでいただきありがとうございました
ポケモン剣盾 コータスの育成論と対策 カバルドンより強い 嵌め殺し陸亀 Yoshiblog
『ポケットモンスター』に登場するキャラクター（モンスター）の一種。 pixivで「サーナイト」のイラストを見る pixivで「サーナイト」の小説を読む pixivで「サーナイト」のイラストを投稿する
